... disjoint.¹

This is explained by the fact that, in the space of all configurations of $n$ disjoint lines in 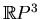$\mathbb{R}P^3$, the configurations containing a line in the plane at infinity form a subset of codimension 2.

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

... space.²

Here are two other problems which are also equivalent: the problem of classifying sets of pairwise transversal two-dimensional subspaces in $\mathbb{R}^4$, with respect to motions under which they remain pairwise transversal two-dimensional subspaces; and the problem of classifying links in the sphere $S^3$ which are made up of great circles on the sphere, with respect to isotopies under which the circles remain disjoint great circles on $S^3$.

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

... configurations.³

We have already encountered this construction. The isotopy join interlacings introduced above (when we treated interlacings of six lines) are essentially the joins of sets of points on a line.

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.